Wave-Particle Duality of Matter

Wave-like Behavior of Matter

In 1925, Louis DeBroglie hypothesized that if light, which everyone thought for so long was a wave, is a particle, then perhaps particles like the electron, proton, and neutron might have wave-like behaviors. He went further and reasoned that since waves are described by their wavelength λ

and particles are described by their momentum, p

then we can relate these two variables by recalling that the Quantum Theory says

Then let's equate these two equations to get the DeBroglie relationship between momentum (a particle property) and wavelength (a wave property)

The first real experimental proof of this relationship came from Davisson and Germer in 1925, who found that electrons will diffract and interfere like waves, just like X-ray photons (light). For example, an electron with a velocity of 5.97 X 10⁶ m/s (mass of an electron =9.11 X 10^-28g) has a wavelength of:

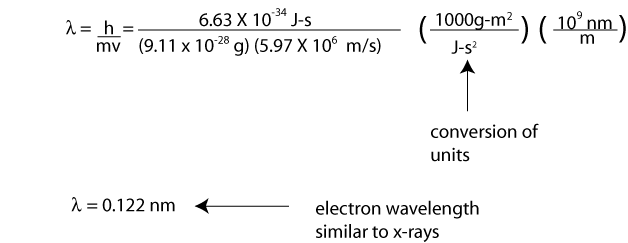
So, matter and light are composed of particles that have wave-like properties. The wave-like behavior is only observed on the subatomic length scales where the masses are small enough for the wavelengths to be detectable.

Quantized States

You may be wondering how does all this about wave-particle duality relates to the electronic structure of the atom. To begin answering that question let's look at an analogy between the standing waves I can set up with a vibrating string, and an electron trapped between two walls.

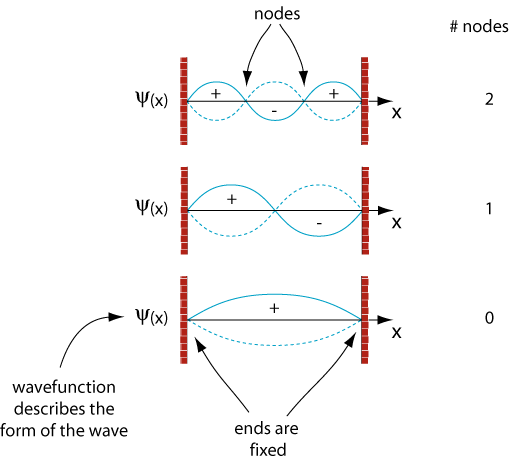
An important property of standing waves is you can't have any frequency you want because ends are fixed. When the ends are fixed only certain discrete wavelengths (frequencies) are allowed (which depend on the length of the string).

nodes are where the wave function changes from positive to negative (ends don't count!). The lowest frequency is called the fundamental or first harmonic and has no nodes. The next frequency is the second harmonic and has one node; it is twice the fundamental frequency. You can keep going, increasing the number of nodes, and increasing the frequency in multiples of the fundamental frequency.

Now we use the analogy of the standing waves of a string because the wavefunction of an electron trapped between two walls would in the same way be constrained to be a standing wave with only discretely allowed wavelengths. That is, an electron trapped between two walls has its wavelength determined by the distance between the walls and the number of nodes.

As in the case of the photon, the energy of the trapped electron is proportional to its frequency. The lowest energy is called the ground state and it has the fundamental frequency. The higher energy states are called the excited states and occur at harmonics (multiples) of the fundamental frequency.

As you can see, the energy states of the trapped electron, just like its frequency, are discrete or quantized. Quantized means that there are only certain "allowed" energy levels or frequencies, and nothing in between. Trapped electrons have quantized energy levels for the same reason that the standing wavelengths we set up with our string are quantized...hence the name Quantum Theory.

What is the physical reality behind this wavefunction?

So we have this picture where electrons, protons, neutrons, and photons are all particles with a wave-like behavior that causes them to constructively and destructively interfere with themselves, and to set up standing waves when confined within boundaries. But what is the physical meaning of this wavefunction that is associated with these subatomic particles?

One interpretation is that the square of the wave function tells us the probability of finding an electron at that point x in space.

So if we square the wavefunctions above we can obtain the probability of finding a trapped electron between two walls as a function of position and the state of the wavefunction (i.e., the number of nodes in its standing wave).

Notice how the probability equals zero at the nodes, since the wave function is always zero at those points. n, the number of nodes plus one, is called the principal quantum number since it fully describes (labels) each state in this one dimensional example.