As we learned earlier the most fundamental building blocks of matter for chemists are atoms (that is, the elements you see in the periodic table). We also learned that atoms can combine with other atoms by chemical bonding to form molecules. Recall that the process where a molecule is transformed into a different molecule is called a chemical change. This process of chemical change is represented by a chemical reaction. For example,
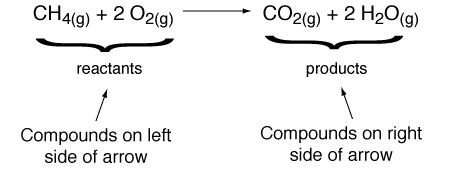
It is important to keep in mind that while in a chemical reaction molecules are destroyed and created by breaking and forming chemical bonds, atoms are neither created nor destroyed in a chemical reaction. In other words - there must be the same number of each type of atom on the product and reactant sides of the arrow. Making sure that this rule is obeyed is called "balancing the chemical equation". In the above example the equation would be unbalanced without the 2 in front of the O2 and H2O. We can make a table to confirm that the number of atoms on each side of the arrow are the same:
| Reactants | Products | Balanced? |
|---|---|---|
| 1 C | 1 C | yes |
| 4 H | 4 H | yes |
| 4 O | 4 O | yes |
Notice that we never change the chemical formula of any product or reactant when trying to balance a chemical equation.
A balanced equation is essential to known the stoichiometry for the chemical reaction.
Stoichiometry - Relationship between quantities of matter that participate in chemical reactions.
-or-
How much of this, plus how much of that gives how much of something else?
Let's consider the following example of water being converted into hydrogen and oxygen gas using a Hoffman Apparatus:
2 H2O(l) → 2 H2(g) + O2(g)
The balanced chemical equation tells us that two molecules of water in the liquid state react to form two molecules of H2 in the gas state and one molecule of O2 in the gas state. The Hoffman apparatus allows us to trap the gaseous H2(g) and gaseous O2(g) in separate volumes. By examining these volumes and using Avogadro's law we can see the stoichiometry of the chemical equation.
Avogadro's Law: The volume of a gas is directly proportional to the number of molecules in the gas.
In this example there is twice the amount of H2 gas and O2 gas, as predicted by the stoichiometry of the chemical equation. These integer relationships between gas volumes were some of the earliest proof we had that matter existed in discrete packages called atoms and molecules.
Just as any good chef wants to add together the correct amount of each ingredient for the best recipe so does the chemist also want to add together the correct number of molecules called for by a chemical reaction to produce the desired product. For example, to separate water molecules into H2 and O2 gas our Hoffmann apparatus had to put electrical energy into the reaction. We could have written the chemical reaction for this as
2 H2O(l) + 571.6 kJ → 2 H2(g) + O2(g)
That is, we use 2 moles of H2O and 571.6 kJ of energy to make 2 moles of H2 gas and 1 mole of O2 gas.
Now let's say we want to do the reverse, that is, convert H2 gas and O2 gas into water and energy.
2 H2(g) + O2(g) → 2 H2O(l) + 571.6 kJ
The stoichiometry tells us that combining two moles of H2(g) with one mole of O2(g) will give 2 moles of H2O(l) plus 571.6 kJ of energy.
So, we take a balloon that is filled with only H2 gas. There will be some O2 molecules in the air outside the balloon but we will take no care in making sure that there will be stoichiometric amounts of H2 and O2 gases together when we start the reaction.

Next let's take a balloon that is filled with the correct amount of H2 and O2 gases according to the stoichiometric ratio of 2:1 from the chemical equation.

When I start the reaction in the H2 only balloon I won't get the maximum abount of H2O and energy (released in part as sound) because the stoichiometry isn't in the best ratio.
When I start the reaction in the 2:1 H2 to O2 balloon I expect to get the maximum amount of H2O and energy (released in part as sound).
Thanks to Amadeus Avogadro we know the volume of a gas is directly proportional to the number of molecules in the gas (or the number of moles). Thus to get the correct stoichiometry when working with gases we can simply adjust the volume of each gas to match the stoichiometry of the reaction (as I did with the second balloon). Generally, however, equal volumes of different compounds in the solid and liquid state do not necessarily contain the same number of molecules, atoms or moles.
When working with solids or liquids it would be nice to have a scale or device that easily measures the number of moles. In practice, however, the starting point for chemists is the mass of the reactants, not the moles. Therefore we need to convert between mass (easily measured) and moles (not so easily measured). Let's look at a few examples.
What mass of oxygen will react with 96.1 grams of propane?
First, write the balanced reaction:
C3H3(g) + 5 O2(g) → 3 CO2(g) + 4 H2O(g)
Second, convert the mass to moles. Note that the molecular weight of propane is 44.1 g/mole.

Baking soda (NaHCO3) is used as an antacid. It neutralize excess HCl secreted by the stomach.
NaHCO3 + HCl(aq) → NaCl(aq) + H2O(l) + CO2(aq)
How many moles of HCl are neutralized per gram of NaHCO3?
We set this problem up similar to the previous one:

Now try this one at home. Milk of Magnesia (Mg(OH)2) is also an antacid, and the chemical reaction is
Mg(OH)2(s) + 2HCl(aq) → 2 H2O(l) + MgCl2(aq)
Try this one on your own: Which neutralizes more HCl per gram, NaHCO3 or Mg(OH)2?

